Solved Problem on Fluid Mechanics

A solid float in the water with 1/8 of its volume immersed. The same body floats in oil with 1/6 of its volume immersed. Determine the ratio of the density of oil ρ_o to the density of water ρ_w.

Problem data:

Fraction of the volume of the body immersed in water: \( \dfrac{1}{8}V \);
Fraction of the volume of the body immersed in oil: \( \dfrac{1}{6}V \).

Problem diagram:

When the body is immersed in water \( \dfrac{1}{8} \) of its volume, it sinks displacing a mass of water equal to the mass of the entire body, the buoyant force and the gravitational force are in equilibrium (Figure 1).

Figure 1

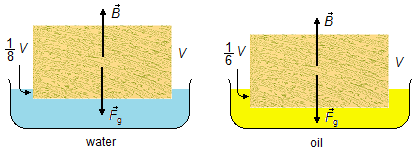
When the body is immersed in oil \( \dfrac{1}{6} \) of its volume it sinks displacing a mass of oil equal to the mass of the whole body, the buoyant force and the gravitational force are in equilibrium.

Solution

As the body is in equilibrium, the gravitational force \( {\vec F}_{g} \) and the force of buoyancy \( \vec B \) cancel out.

\[ \begin{gather} B=F_{g} \tag{I} \end{gather} \]

The buoyant force is given by

\[ \begin{gather} \bbox[#99CCFF,10px] {B=m_{L}g} \tag{II} \end{gather} \]

where m_L is the mass of water displaced.
The density of a body is given by

\[ \begin{gather} \bbox[#99CCFF,10px] {\rho=\frac{m}{V}} \tag{III} \end{gather} \]

\[ \begin{gather} m=\rho V \tag{IV} \end{gather} \]

Using expression (IV) the mass of liquid displaced will be

\[ \begin{gather} m_{L}=\rho_{L}V_{L} \tag{V} \end{gather} \]

substituting expression (V) into expression (II)

\[ \begin{gather} B=\rho_{L}V_{L}g \tag{VI} \end{gather} \]

Body floating in water:

Substituting expression (VI) into expression (I), and using the problem condition that the volume of water displaced V_w is \( \dfrac{1}{8} \) of the total body volume

\[ \begin{gather} B=\rho_{w}\left(\frac{1}{8}V\right)g \tag{VII} \end{gather} \]

substituting expression (VII) into expression (I)

\[ \begin{gather} \frac{1}{8}\rho_{w}Vg=F_{g} \tag{VIII} \end{gather} \]

Body floating in oil:

Substituting expression (VI) into expression (I), and using the problem condition that the volume of oil displaced V_o is \( \dfrac{1}{6} \) of the total body volume

\[ \begin{gather} B=\rho_{o}\left(\frac{1}{6}V\right)g \tag{IX} \end{gather} \]

substituting expression (IX) into expression (I)

\[ \begin{gather} \frac{1}{6}\rho_{o}Vg=F_{g} \tag{X} \end{gather} \]

Equating the expressions (VIII) and (X)

\[ \frac{1}{8}\rho_{w}\cancel{V}\cancel{g}=\frac{1}{6}\rho_{o}\cancel{V}\cancel{g} \]

canceling the volume V and acceleration due to gravity g on both sides of the equation

\[ \begin{gather} \frac{1}{8}\rho_{w}=\frac{1}{6}\rho_{o}\\ \frac{\rho_{o}}{\rho_{w}}=\frac{6}{8} \end{gather} \]

\[ \bbox[#FFCCCC,10px] {\frac{\rho_{o}}{\rho_{w}}=\frac{3}{4}} \]

Note: Oil density will be 0.75 of water density or 75% of water density (less dense). The body sinks deeper in oil because it is less dense than water, therefore, it produces less buoyant force.

Fisicaexe - Physics Solved Problems by Elcio Brandani Mondadori is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License .