Solved Problem on Magnetic Field
advertisement   



Two circular loops E1 and E2, concentric and coplanar with radii R1=10π cm e R2=2,5π cm carry currents i1 e i2, shown in the figure. The current i1 = 10 A and \( \mu_{0}=4\pi \times10^{-7}\;\frac{\text{T.m}}{\text{A}} \).
a) Calculate the vector of the magnetic field created by the current i1 in the center O; b) Determine the current i2 so that the resultant magnetic field in the center will be zero.


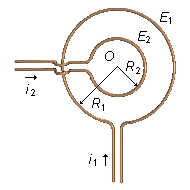
Problem data:
  • Radius of loop 1:    R1 = 10 π cm;
  • Current in the loop 1:    i1 = 10 A;
  • Radius of loop 2:    R2 = 2.5 π cm;
  • Vacuum permeability:    \( \mu_{0}=4\pi \times 10^{-7}\;\frac{\text{T.m}}{\text{A}} \).
Solution

First, we must convert the units of the radii of the loops given in centimeters (cm) to meters (m)) used in the International System of Units (S.I.).
\[ \begin{gather} R_{1}=10\pi\;\cancel{\text{cm}}\times \frac{1\;\text{m}}{100\;\cancel{\text{cm}}}=0.1\pi\;\text{m}=10^{-1}\pi \;\text{m}\\[10pt] R_{2}=2.5\pi\;\cancel{\text{cm}}\times \frac{1\;\text{m}}{100\;\cancel{\text{cm}}}=0.025\pi \;\text{m}=2.5\pi\times 10^{-2}\;\text{m} \end{gather} \]
a) The field created by current i1 in the center of the loop E1 can be obtained by applying the right-hand rule. Pointing the thumb in the direction of the current i1 the other fingers will indicate the direction of the field, which in this case it is perpendicular to the plane of the loop and pointing into the page (Figure 1).
The magnitude of the magnetic field B is given by
\[ \begin{gather} \bbox[#99CCFF,10px] {B=\frac{\mu_{0}}{2}\frac{i}{r}} \tag{I} \end{gather} \]
Figure 1

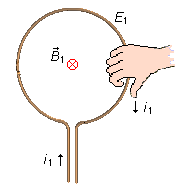
For the magnetic field B1, applying the expression (I), we have
\[ \begin{gather} B_{1}=\frac{\mu_{0}}{2}\frac{i_{1}}{r_{1}}\\ B_{1}=\frac{4\pi \times 10^{-7}}{2}\times \frac{10}{10^{-1}\pi}\\ B_{1}=2\times 10^{-7}\times 10\times 10\\ B_{1}=2\times 10^{-5}\;\text{T} \tag{II} \end{gather} \]
thus the magnetic field at the center of the loop can be characterized by

Magnitude: B1 = 2× 10−5 T ;
Direction: perpendicular to the plane of the loop and pointing into the page .

b) Applying the right-hand rule the loop E2, ointing the thumb in the direction of the current the other fingers indicate that the magnetic field will be perpendicular to the loop and pointing out of the page (Figure 2).
Figure 2

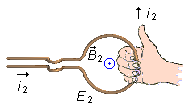
For the magnetic field B2, we have
\[ \begin{gather} B_{2}=\frac{\mu_{0}}{2}\frac{i_{2}}{r_{2}}\\ B_{2}=\frac{4\pi \times 10^{-7}}{2}\times \frac{i_{2}}{2.5\pi\times 10^{-2}}\\ B_{2}=0.8\times 10^{-7}\times 10^{2}\,i_{2}\\ B_{2}=0.8\times 10^{-5}\,i_{2} \tag{III} \end{gather} \]
A view in perspective (Figure 3) shows that the vectors of the magnetic field, B1 and B2 are in the opposite directions, so that the fields to cancel out at the center we must of the condition that their magnitudes are equal
\[ B_{1}=B_{2} \]
equating expressions (II) and (III), we have
\[ \begin{gather} 2\times 10^{-5}=0.8\times 10^{-5}\,i_{2}\\ i_{2}=\frac{2\times 10^{-5}}{0.8\times 10^{-5}} \end{gather} \]

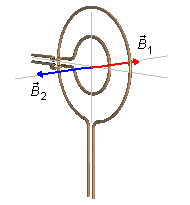
Figure 3
\[ \bbox[#FFCCCC,10px] {i_{2}=2.5\;\text{A}} \]
advertisement   

Creative Commons License
Fisicaexe - Physics Solved Problems by Elcio Brandani Mondadori is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License .