Solved Problem on Coulomb's Law

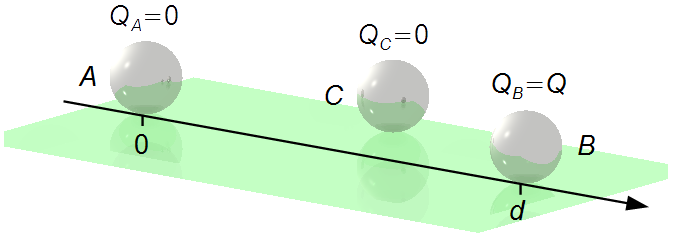
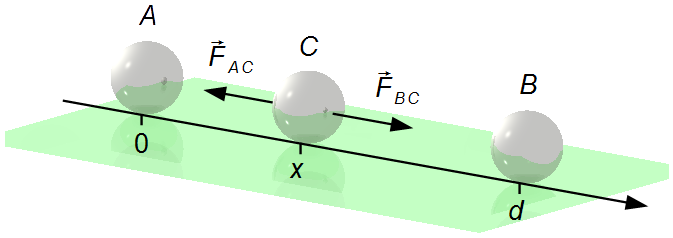
Two identical spheres, A and B, are fixed on a flat, horizontal glass sheet at a distance d from each other. Sphere A is initially neutral, and B is charged. A third sphere C, identical to the first two and initially neutral, is placed in contact with B and then with A. Determine at what distance x from sphere A, on the straight line AB, should sphere C be placed so that it remains in equilibrium.

Problem data:

Charge of sphere A: Q_A = 0;
Charge of sphere B: Q_B = Q;
Charge of sphere C: Q_C = 0;
Distance between spheres A and B: d.

Solution

We use Q for the initial charge of sphere B, and a reference frame with an origin in A and oriented to B. Sphere B is at a distance d from sphere A (Figure 1). First, we calculate the charge that the other spheres will acquire by contact.

Figure 1

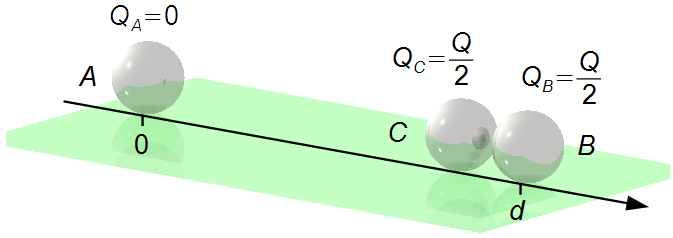
We put spheres B and C in contact, the charge of B will be distributed equally over the two spheres (Figure 2)

\[ \begin{gather} Q_{B}=Q_{C}=\frac{Q_{B}+Q_{C}}{2}=\frac{Q+0}{2}=\frac{Q}{2} \end{gather} \]

Figure 2

Now sphere C, with a charge equal to \( \frac{Q}{2} \), is placed in contact with sphere A with a charge equal to zero, and the charge of C will be distributed over both spheres (Figure 3).

Figure 3

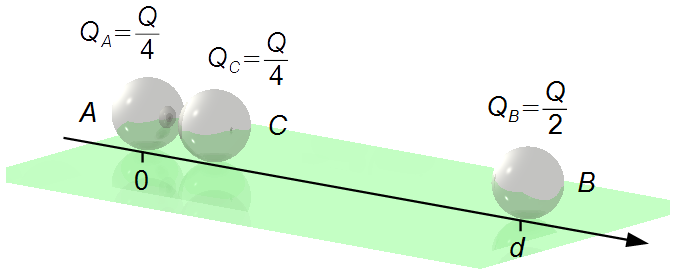
\[ \begin{gather} Q_{A}=Q_{C}=\frac{Q_{A}+Q_{C}}{2}=\frac{0+\dfrac{Q}{2}}{2}=\frac{Q}{2}\times\frac{1}{2}=\frac{Q}{4} \end{gather} \]

In the final situation, spheres A and C have charges \( Q_{A}=Q_{C}=\frac{Q}{4} \), and sphere B has charge \( Q_{B}=\frac{Q}{2} \). Sphere C should be placed at a point x between A and B to remain in equilibrium (Figure 4).

Figure 4

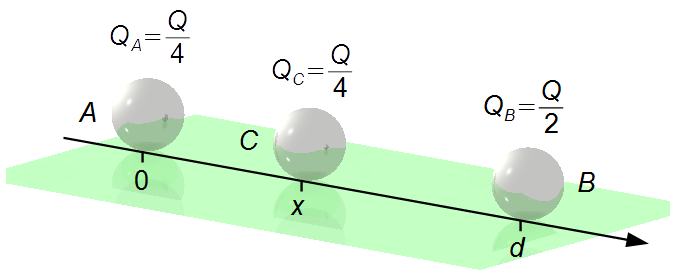
For it to be in equilibrium, the force acting between spheres A and C must be equal to the force acting between B and C, so that the resultant of the forces is equal to zero (Figure 5)

\[ \begin{gather} {\vec{F}}_{AC}={\vec{F}}_{BC} \tag{I} \end{gather} \]

According to Coulomb's Law, the electric force is given, in magnitude, by

Figure 5

\[ \begin{gather} \bbox[#99CCFF,10px] {F_{E}=k_{0}\frac{|Q_{1}||Q_{2}|}{r^{2}}} \tag{II} \end{gather} \]

Applying expression (II) to spheres A and C

\[ \begin{gather} F_{AC}=k_{0}\frac{Q_{A}Q_{C}}{x^{2}}\\[5pt] F_{AC}=k_{0}\frac{\dfrac{Q}{4}\dfrac{Q}{4}}{x^{2}}\\[5pt] F_{AC}=k_{0}\frac{Q^{2}}{16x^{2}} \tag{III} \end{gather} \]

For spheres B and C, the distance between them will be r = (d − x), substituting the data in (II) for this situation

\[ \begin{gather} F_{BC}=k_{0}\frac{Q_{B}Q_{C}}{(d-x)^{2}}\\[5pt] F_{BC}=k_{0}\frac{\dfrac{Q}{2}\dfrac{Q}{4}}{(d-x)^{2}}\\[5pt] F_{BC}=k_{0}\frac{Q^{2}}{8(d-x)^{2}} \tag{IV} \end{gather} \]

Applying condition (I) to expressions (III) and (IV)

\[ \begin{gather} \cancel{k_{0}}\frac{\cancel{Q^{2}}}{\cancelto{2}{16}x^{2}}=\cancel{k_{0}}\frac{\cancel{Q^{2}}}{\cancel{8}(d-x)^{2}}\\[5pt] \frac{1}{2x^{2}}=\frac{1}{(d-x)^{2}}\\[5pt] (d-x)^{2}=2x^{2}\\[5pt] d^{2}-2dx+x^{2}=2x^{2}\\[5pt] 2x^{2}-x^{2}+2dx-d^{2}=0\\[5pt] x^{2}+2dx-d^{2}=0 \end{gather} \]

This is a Quadratic Equation in x.

Solution of the Quadratic Equation \( x^{2}+2dx-d^{2}=0 \)

\[ \begin{gather} \Delta =b^{2}-4ac=\left(2d\right)^{2}-4\times 1\times (-d^{2})=4d^{2}+4d^{2}=8d^{2}\\[10pt] x=\frac{-b\pm\sqrt{\Delta }}{2a}=\frac{-2d\pm \sqrt{8d^{2}}}{2\times 1}=\frac{-2d\pm2d\sqrt{2\;}}{2} \end{gather} \]

the two roots of the equation will be

\[ \begin{gather} x_{1}=d(\sqrt{2\;}-1)\\ \qquad \mathrm{e}\\ x_{2}=-d(\sqrt{2\;}-1) \end{gather} \]

The distance d is greater than zero, and the term in parentheses, \( (\sqrt{2 \;}-1)\approx 0.4142 \), is greater than zero. So the first root is worth approximately 0.41d, therefore it is between spheres A and B as the statement asks; the second root has a negative sign, so it is to the left of sphere A, on the negative side of the reference frame (Figure 1) and can be neglected.

\[ \begin{gather} \bbox[#FFCCCC,10px] {x_{1}=d(\sqrt{2 \;}-1)} \end{gather} \]

Fisicaexe - Physics Solved Problems by Elcio Brandani Mondadori is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License .