Exercício Resolvido de Funções Complexas
publicidade
c) \( \displaystyle (1+i)^{i} \)
Usando a expressão
\[ \bbox[#99CCFF,10px]
{u^{v}=\operatorname{e}^{v\;\text{Ln}u}}
\]
\[
\begin{gather}
(1+i)^{i}=\operatorname{e}^{i\;\operatorname{Ln}(1+i)} \tag{I}
\end{gather}
\]
A função multivalente do logaritmo é dada por
\[
\begin{gather}
\bbox[#99CCFF,10px]
{\operatorname{Ln}z=\ln |z|+i(\operatorname{arg}(z)+2k\pi)} \tag{II}
\end{gather}
\]
O argumento do logaritmo é o número complexo da forma
\[
u=1+i
\]
O módulo é dado por
\[ \bbox[#99CCFF,10px]
{|z|=\sqrt{x^{2}+y^{2}}}
\]
\[
\begin{gather}
|u|=\sqrt{1^{2}+1^{2}\;}\\
|u|=\sqrt{2\;} \tag{III}
\end{gather}
\]
O argumento é dado por
\[ \bbox[#99CCFF,10px]
{\theta=\operatorname{arg}(z)=\operatorname{arctg}\left(\frac{y}{x}\right)}
\]
\[
\begin{align}
\theta &=\operatorname{arg}(u)=\operatorname{arctg}\left(\frac{y}{x}\right)=\\
&=\operatorname{arctg}\left(\frac{1}{1}\right)=\operatorname{arctg}(1)=\frac{\pi}{4} \tag{IV}
\end{align}
\]
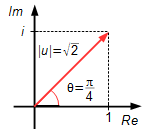
Gráfico 1
substituindo os valores (III) e (IV) na expressão (II)
\[
\begin{gather}
\operatorname{Ln}(1+i)=\ln \left(\sqrt{2\;}\right)+i\left(\frac{\pi}{4}+2k\pi\right) \tag{V}
\end{gather}
\]
substituindo a expressão (V) na expressão (I)
\[
\begin{gather}
(1+i)^{i}=\operatorname{e}^{i\;\left[\ln\left(\sqrt{2\;}\right)+i\left(\frac{\pi}{4}+2k\pi\right)\right]}\\[5pt]
(1+i)^{i}=\operatorname{e}^{i\;\ln\left(\sqrt{2\;}\right)+\mathit{i.i}\left(\frac{\pi}{4}+2k\pi\right)}\\[5pt]
(1+i)^{i}=\operatorname{e}^{i\;\ln\left(\sqrt{2\;}\right)+i^{2}\left(\frac{\pi}{4}+2k\pi\right)}\\[5pt]
(1+i)^{i}=\operatorname{e}^{i\;\ln\left(\sqrt{2\;}\right)-\left(\frac{\pi}{4}+2k\pi\right)}\\[5pt]
(1+i)^{i}=\operatorname{e}^{i\;\ln\left(\sqrt{2\;}\right)-\frac{\pi}{4}-2k\pi}
\end{gather}
\]
\[
\begin{gathered}
(1+i)^{i}=\operatorname{e}^{i\;\ln\left(\sqrt{2\;}\right)-\frac{\pi}{4}+2k\pi}\\[5pt]
\qquad \qquad \quad\ (1+i)^{i}=\operatorname{e}^{i\;\ln\left(\sqrt{2\;}\right)+\left(2k-\frac{1}{4}\right)\;\pi}\quad ,\quad k\in\mathbb{Z}
\end{gathered}
\]
\[
\begin{gather}
(1+i)^{i}=\operatorname{e}^{i\;\ln\left(\sqrt{2\;}\right)+\left(2k-\frac{1}{4}\right)\;\pi}\\
(1+i)^{i}=\operatorname{e}^{i\;\ln\left(\sqrt{2\;}\right)}.\operatorname{e}^{\left(2k-\frac{1}{4}\right)\;\pi}
\end{gather}
\]
Aplicando a Fórmula de Euler
\[ \bbox[#99CCFF,10px]
{\operatorname{e}^{i\theta}=\cos \theta +i\operatorname{sen}\theta}
\]
\[
\begin{gather}
(1+i)^{i}=\operatorname{e}^{\left(2k-\frac{1}{4}\right)\;\pi}\left[\cos \left(\ln\left(2^{1/2}\right)\right)+i\operatorname{sen}\left(\ln\left(2^{1/2}\right)\right)\right]\\[5pt]
(1+i)^{i}=\operatorname{e}^{\left(2k-\frac{1}{4}\right)\;\pi}\left[\cos \left(\frac{1}{2}\ln2\right)+i\operatorname{sen}\left(\frac{1}{2}\ln2\right)\right]
\end{gather}
\]
\[ \bbox[#FFCCCC,10px]
{(1+i)^{i}=\operatorname{e}^{\left(2k-\frac{1}{4}\right)\;\pi}\left(\cos\frac{\ln 2}{2}+i\operatorname{sen}\frac{\ln 2}{2}\right)}
\]
publicidade

Fisicaexe - Exercícios Resolvidos de Física de Elcio Brandani Mondadori está licenciado com uma Licença Creative Commons - Atribuição-NãoComercial-Compartilha Igual 4.0 Internacional .