Solved Problem on Kinematics
advertisement
Two particles run perpendicular to each other trajectories that intersect at a common origin. The particles leave at the same time from points x0 and y0 toward the origin, both with the same constant acceleration in magnitude. Calculate:
a) After how long after they leave they will be at the smallest distance; b) What is the smallest distance.
Problem data:
- Initial position of the particle 1: x0;
- Initial speed of the particle 1: v0x = 0;
- Acceleration of the particle 1: −a;
- Initial position of the particle 2: y0;
- Initial speed of the particle 2: v0y = 0;
- Acceleration of the particle 2: −a;
Let's assume the starting points of the bodies are positive (x0 > 0 and
y0 > 0). As they move toward the origin their accelerations are in the opposite
direction of the orientation of the trajectories and are negative (a < 0).
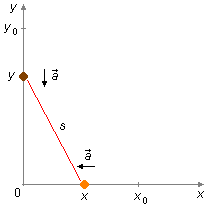
At a certain moment, they occupy points x and y such that the distance d between them are the smallest (Figure 1).
At a certain moment, they occupy points x and y such that the distance d between them are the smallest (Figure 1).
a) The equation of displacement as a function of time with constant acceleration is given by
\[ \bbox[#99CCFF,10px]
{x(t)=x_{0}+v_{0}(t-t_{0})+\frac{a}{2}(t-t_{0})^{2}}
\]
writing the equations for the two particles at t0 = 0
\[
\begin{gather}
x=x_{0}+v_{0x}t-\frac{a}{2}t^{2}\\
x=x_{0}+0.t-\frac{a}{2}t^{2}\\
x=x_{0}-\frac{a}{2}t^{2} \tag{I}
\end{gather}
\]
\[
\begin{gather}
y=y_{0}+v_{0y}t-\frac{a}{2}t^{2}\\
y=y_{0}+0.t-\frac{a}{2}t^{2}\\
y=y_{0}-\frac{a}{2}t^{2} \tag{II}
\end{gather}
\]
From Figure 1, we calculate the distance between the particles using the Pythagorean Theorem
\[
\begin{gather}
s^{2}=x^{2}+y^{2} \tag{III}
\end{gather}
\]
substituting the expressions (I) and (II) into expression (III)
\[
\begin{gather}
s=\left[\left(x_{0}-\frac{a}{2}t^{2}\right)^{2}+\left(y_{0}-\frac{a}{2}t^{2}\right)^{2}\right]^{\;\frac{1}{2}} \tag{IV}
\end{gather}
\]
To find the instant when the distance is the smallest, we take the derivative of expression (IV) with
respect to time and equal to zero.
Differentiation of
\( \displaystyle s=\left[\left(x_{0}-\frac{a}{2}t^{2}\right)^{2}+\left(y_{0}-\frac{a}{2}t^{2}\right)^{2}\right]^{\;\frac{1}{2}} \)
The function s(t) is a composite function whose derivative is given by the chain rule
\[ \displaystyle s=\left[\left(x_{0}-\frac{a}{2}t^{2}\right)^{2}+\left(y_{0}-\frac{a}{2}t^{2}\right)^{2}\right]^{\;\frac{1}{2}} \]
The function s(t) is a composite function whose derivative is given by the chain rule
\[
\begin{gather}
\frac{ds[g(t)]}{dt}=\frac{ds}{dg}\;\frac{dg}{dt} \tag{V}
\end{gather}
\]
with
\( s(g)=g^{\frac{\;1}{2}} \)
and
\( g(t)=\left(x_{0}-\dfrac{a}{2}t^{2}\right)^{2}+\left(y_{0}-\dfrac{a}{2}t^{2}\right)^{2} \text{,} \)
\[ g(t)=\left(x_{0}-\dfrac{a}{2}t^{2}\right)^{2}+\left(y_{0}-\dfrac{a}{2}t^{2}\right)^{2} \]
the differentiation will be
\[
\begin{align}
&\frac{ds}{dg}=\frac{1}{2}g^{\frac{1}{2}-1}=\frac{1}{2}g^{\frac{1-2}{2}}=\frac{1}{2}g^{-{\frac{1}{2}}}=\frac{1}{2}\left[\left(x_{0}-\frac{a}{2}t^{2}\right)^{2}+\left(y_{0}-\frac{a}{2}t^{2}\right)^{2}\right]^{-{\frac{1}{2}}} \tag{VI}\\[10pt]
&\frac{dg}{dt}=\frac{d}{dt}\left[\left(x_{0}-\frac{a}{2}t^{2}\right)^{2}+\left(y_{0}-\frac{a}{2}t^{2}\right)^{2}\right] \tag{VII}
\end{align}
\]
substituting the expressions (VI) and (VII) into expression (V)
\[
\begin{gather}
\frac{ds[g(t)]}{dt}=\frac{1}{2}\left[\left(x_{0}-\frac{a}{2}t^{2}\right)^{2}+\left(y_{0}-\frac{a}{2}t^{2}\right)^{2}\right]^{-{\frac{1}{2}}}\;\frac{d}{dt}\left[\left(x_{0}-\frac{a}{2}t^{2}\right)^{2}+\left(y_{0}-\frac{a}{2}t^{2}\right)^{2}\right] \tag{VIII}
\end{gather}
\]
the derivative of a sum is the sum of the derivatives
\[
\begin{gather}
\frac{dg}{dt}=\frac{d}{dt}\left(x_{0}-\frac{a}{2}t^{2}\right)^{2}+\frac{d}{dt}\left(y_{0}-\frac{a}{2}t^{2}\right)^{2} \tag{IX}
\end{gather}
\]
substituting the expression (IX) into expression (VIII)
\[
\begin{gather}
\frac{ds[g(t)]}{dt}=\frac{1}{2}\left[\left(x_{0}-\frac{a}{2}t^{2}\right)^{2}+\left(y_{0}-\frac{a}{2}t^{2}\right)^{2}\right]^{-{\frac{1}{2}}}\;\left[\frac{d}{dt}\left(x_{0}-\frac{a}{2}t^{2}\right)^{2}+\frac{d}{dt}\left(y_{0}-\frac{a}{2}t^{2}\right)^{2}\right] \tag{X}
\end{gather}
\]
each of the functions in the parentheses is a composite function, whose derivative is given by the
chain rule
\[
\begin{gather}
\frac{dh[u(t)]}{dt}=\frac{dh}{du}\;\frac{du}{dt} \tag{XI}
\end{gather}
\]
applying the expression (XI) to the term
\( \displaystyle \left(x_{0}-\frac{a}{2}t^{2}\right)^{2} \)
of the expression (X), with
\( h(u)=u^{2} \)
and
\( \displaystyle u(t)=\left(x_{0}-\frac{a}{2}t^{2}\right) \),
thus derivatives are
\[
\begin{gather}
\frac{dh}{du}=2u^{2-1}=2u \tag{XII}
\end{gather}
\]
\[
\begin{gather}
\frac{du}{dt}=\left(0-2\frac{a}{2}t^{2-1}\right)=-at \tag{XIII}
\end{gather}
\]
substituting the expressions (XII) and (XIII) into expression (XI)
\[
\begin{gather}
\frac{du[h(t)]}{dt}=-2\left(x_{0}-\frac{a}{2}t^{2}\right)at \tag{XIV}
\end{gather}
\]
again using the chain rule
\[
\begin{gather}
\frac{df[v(t)]}{dt}=\frac{df}{dv}\;\frac{dv}{dt} \tag{XV}
\end{gather}
\]
applying the expression (XV) to the term
\( \displaystyle \left(y_{0}-\frac{a}{2}t^{2}\right)^{2} \)
of the expression (X), with
\( f(v)=v^{2} \)
and
\( \displaystyle v(t)=\left(y_{0}-\frac{a}{2}t^{2}\right) \),
thus derivatives are
\[
\begin{align}
&\frac{df}{dv}=2v^{2-1}=2v \tag{XVI} \\[10pt]
&\frac{dv}{dt}=\left(0-2\frac{a}{2}t^{2-1}\right)=-at \tag{XVII}
\end{align}
\]
substituting the expressions (XVI) and (XVII) into expression (XV)
\[
\begin{gather}
\frac{df[v(t)]}{dt}=-2\left(y_{0}-\frac{a}{2}t^{2}\right)at \tag{XVIII}
\end{gather}
\]
substituting expressions (XIV) and (XVIII) into expression (X)
\[
\begin{gather}
\frac{ds}{dt}=\frac{1}{2}\left[\left(x_{0}-\dfrac{a}{2}t^{2}\right)^{2}+\left(y_{0}-\dfrac{a}{2}t^{2}\right)^{2}\right]^{-{\frac{1}{2}}}\;\left[-2\left(x_{0}-\dfrac{a}{2}t^{2}\right)at-2\left(y_{0}-\dfrac{a}{2}t^{2}\right)at\right]\\[5pt]
\frac{ds}{dt}=\frac{1}{\cancel{2}}\frac{(-\cancel{2}at)\;\left[\left(x_{0}-\dfrac{a}{2}t^{2}\right)+\left(y_{0}-\dfrac{a}{2}t^{2}\right)\right]}{\left[\left(x_{0}-\dfrac{a}{2}t^{2}\right)^{2}+\left(y_{0}-\dfrac{a}{2}t^{2}\right)^{2}\right]^{-{\frac{1}{2}}}}\\[5pt]
\frac{ds}{dt}=-{\frac{at\;\left[x_{0}-\dfrac{a}{2}t^{2}+y_{0}-\dfrac{a}{2}t^{2}\right]}{\left[\left(x_{0}-\dfrac{a}{2}t^{2}\right)^{2}+\left(y_{0}-\dfrac{a}{2}t^{2}\right)^{2}\right]^{-{\frac{1}{2}}}}}\\[5pt]
\frac{ds}{dt}=-{\frac{at\;\left[x_{0}+y_{0}-at^{2}\right]}{\left[\left(x_{0}-\dfrac{a}{2}t^{2}\right)^{2}+\left(y_{0}-\dfrac{a}{2}t^{2}\right)^{2}\right]^{-{\frac{1}{2}}}}} \tag{XIX}
\end{gather}
\]
the derivative must be zero \( \left(\frac{ds}{dt}=0\right) \), we have in the expression (V)
\[
\begin{gather}
-{\frac{at\;\left[x_{0}+y_{0}-at^{2}\right]}{\left[\left(x_{0}-\dfrac{a}{2}t^{2}\right)^{2}+\left(y_{0}-\dfrac{a}{2}t^{2}\right)^{2}\right]^{-{\frac{1}{2}}}}}=0\\[5pt]
x_{0}+y_{0}-at^{2}=-{\frac{0}{at}.\left[\left(x_{0}-\dfrac{a}{2}t^{2}\right)^{2}+\left(y_{0}-\dfrac{a}{2}t^{2}\right)^{2}\right]^{-{\frac{1}{2}}}}\\[5pt]
x_{0}+y_{0}-at^{2}=0\\at^{2}=x_{0}+y_{0}\\[5pt]
t^{2}=\frac{x_{0}+y_{0}}{a}
\end{gather}
\]
\[ \bbox[#FFCCCC,10px]
{t=\sqrt{\frac{x_{0}+y_{0}}{a}\;}}
\]
b) The smallest distance is obtained by substituting the value of the time found in item (a) into the expression (IV)
\[
\begin{gather}
s=\left[\left(x_{0}-\frac{a}{2}\left(\sqrt{\frac{x_{0}+y_{0}}{a}\;}\right)^{2}\right)^{2}+\left(y_{0}-\frac{a}{2}\left(\sqrt{\frac{x_{0}+y_{0}}{a}\;}\right)^{2}\right)^{2}\right]^{\;\frac{1}{2}}\\
s=\left[\left(x_{0}-\frac{a}{2}\left(\frac{x_{0}+y_{0}}{a}\right)\right)^{2}+\left(y_{0}-\frac{a}{2}\left(\frac{x_{0}+y_{0}}{a}\right)^{2}\right)^{2}\right]^{\;\frac{1}{2}}\\
s=\left[\left(x_{0}-\frac{x_{0}}{2}-\frac{y_{0}}{2}\right)^{2}+\left(y_{0}-\frac{x_{0}}{2}-\frac{y_{0}}{2}\right)^{2}\right]^{\;\frac{1}{2}}\\
s=\left[\left(\frac{x_{0}}{2}-\frac{y_{0}}{2}\right)^{2}+\left(\frac{y_{0}}{2}-\frac{x_{0}}{2}\right)^{2}\right]^{\;\frac{1}{2}}\\
s=\left[\frac{1}{4}\left(x_{0}-y_{0}\right)^{2}+\frac{1}{4}\left(y_{0}-x_{0}\right)^{2}\right]^{\;\frac{1}{2}}\\
s=\frac{1}{2}\left[x_{0}^{2}-2x_{0}y_{0}+y_{0}^{2}+y_{0}^{2}-2y_{0}x_{0}+x_{0}^{2}\right]^{\;\frac{1}{2}}\\
s=\frac{1}{2}\left[2x_{0}^{2}-4x_{0}y_{0}+2y_{0}^{2}\right]^{\;\frac{1}{2}}\\
s=\frac{\sqrt{2}}{2}\left[x_{0}^{2}-2x_{0}y_{0}+y_{0}^{2}\right]^{\;\frac{1}{2}}\\
s=\frac{\sqrt{2}}{2}\left[\left(x_{0}-y_{0}\right)^{2}\right]^{\;\frac{1}{2}}
\end{gather}
\]
\[ \bbox[#FFCCCC,10px]
{s=\frac{\sqrt{2}}{2}\left(x_{0}-y_{0}\right)}
\]
advertisement

Fisicaexe - Physics Solved Problems by Elcio Brandani Mondadori is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License .